Understanding natural frequency is crucial for designing resilient structures and machines. This guide navigates the complexities of natural frequency, offering practical insights and advanced techniques for ensuring structural integrity and preventing resonance failures.
Natural frequency is the inherent rate at which an object vibrates when disturbed. Every object, from a simple spring to a complex bridge, possesses a natural frequency. When an external force matches this frequency, resonance occurs, amplifying vibrations and potentially leading to catastrophic failure. Accurately predicting and managing natural frequency is paramount for safe and reliable design.
Calculating Natural Frequency: From Simple to Complex
Simple Harmonic Oscillators: The Spring-Mass System
The most basic example is a mass attached to a spring. The natural frequency ( f ) is calculated using:
f = √(k/m) / 2π
Where:
f = Natural frequency (Hz)
k = Spring stiffness (N/m)
m = Mass (kg)
A stiffer spring or a lighter mass results in a higher natural frequency.
Beyond the Basics: Modal Analysis and Finite Element Analysis (FEA)
Real-world structures are far more complex than a simple spring-mass system. For intricate designs, advanced techniques like Modal Analysis and FEA are essential.
Modal Analysis: Identifies all potential natural frequencies (modes) and their corresponding vibration patterns (mode shapes) of a structure. This helps understand how a structure will respond to various dynamic loads.
Finite Element Analysis (FEA): Divides a complex structure into numerous smaller elements, allowing for detailed simulations and precise predictions of natural frequencies and stress distributions under various loading conditions. FEA software like ANSYS, Abaqus, COMSOL, and SimScale are invaluable tools for these analyses.
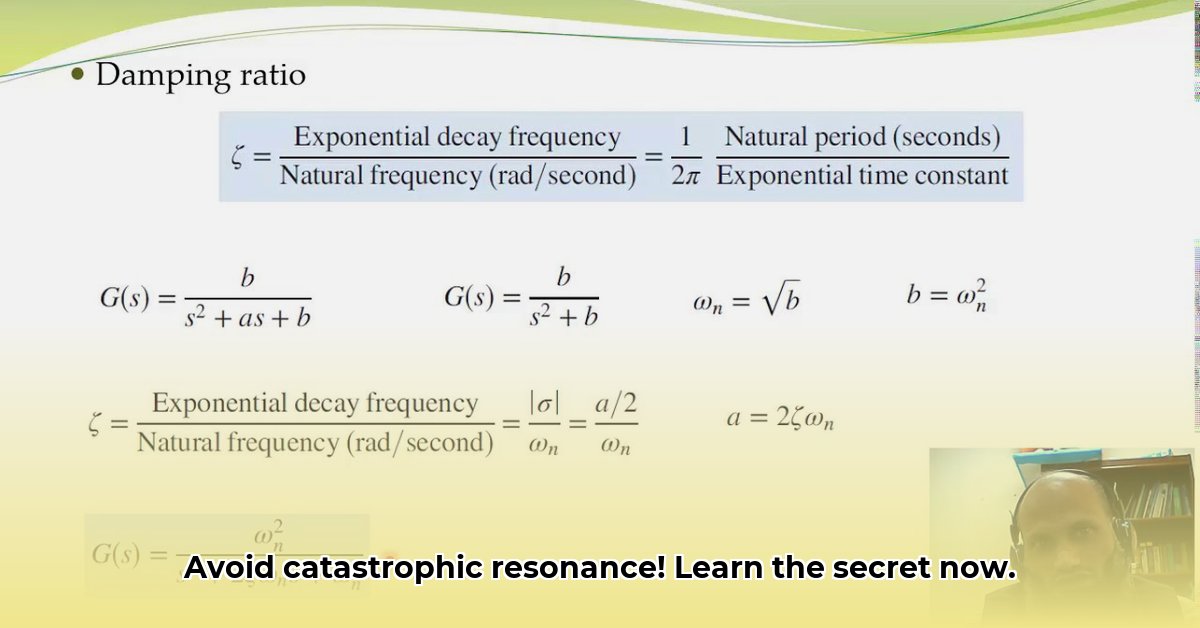
Damping: A Critical Factor
Damping, often overlooked in simplified calculations, plays a crucial role in real-world scenarios. Damping dissipates vibrational energy, reducing the amplitude of oscillations and shifting the resonant frequency. Factors contributing to damping include material properties, friction, and air resistance. Accurately modeling damping is essential for realistic predictions and safe designs.
Practical Steps for Natural Frequency Analysis
System Characterization: Accurately define the system’s geometry, material properties, boundary conditions, and potential external forces.
Method Selection: Choose the appropriate analysis method based on the system’s complexity. Simple formulas suffice for basic systems, while FEA is necessary for complex structures.
Model Validation: Verify the accuracy of the model and input parameters through comparisons with experimental data or established benchmarks.
Damping Considerations: Incorporate damping effects for realistic predictions, especially when dealing with dynamic loads.
Safety Margins: Design with appropriate safety factors to account for uncertainties and potential variations in real-world conditions.
Industry Standards: Adhere to relevant industry codes and standards for specific applications, such as seismic design or aerospace engineering.
Advanced Techniques and Applications
Complex Mechanical Systems
FEA is crucial for analyzing complex mechanical systems with intricate geometries and multiple components. It allows for detailed simulations of dynamic behavior under various loading conditions, aiding in optimizing designs for vibration control and preventing resonance-induced failures.
Seismic Design
In earthquake-prone regions, accurately determining a structure’s natural frequency is critical. Seismic design incorporates advanced techniques to ensure that a building’s natural frequency does not coincide with the dominant frequencies of ground motion during an earthquake. This minimizes the risk of resonance and prevents catastrophic collapse.
Ongoing Research and Future Directions
Research continues to refine natural frequency analysis techniques. Advanced methods like nonlinear FEA, stochastic analysis, and machine learning are being employed to address complex systems and uncertainties in material properties and loading conditions. These advancements contribute to more robust and reliable designs.
Hello, My love for writing is rivaled only by my passion for in-depth research. While many may skim the surface, I dive deep, seeking the hidden gems of information that give a story texture and depth. Every word I write is backed by hours of exploration, ensuring that my work is not just compelling, but also meticulously accurate. Join me on a journey where every piece is a deep dive into the realms of knowledge and storytelling.
